
Then we need to shade the areas between the curve and the horizontal axis to visualise the solution. Thereafter, given that we know that the curve will be ∪ shaped, we can sketch the graph by connecting the points x1 and x2 and extending our curve toward infinity. The first thing we need to do is to sketch the axis and define on the horizontal axis ( x axis) the position of the points x1 and x2. Here I am using a computer program, but I will lay out the underlying thinking as I go along. In our case the sign of a is positive ( a = 2 ) thus our curve is ∪ shaped.Ħ) Now things become even trickier as we need to sketch the graph. They are called roots.ĥ) Things get a bit harder now as we need to remember that the orientation of the parabola is given by the sign of the a term.
By substituting into the quadratic formula, we obtain:Ĥ) By solving two equations we obtain the two points where the graph crosses the horizontal axis ( x axis). Our aim is to sketch the graph of a parabola, which is a curve with determined properties, to obtain a mathematical solution from our plot.ģ) At this point we need to remember that a quadratic equation has the form y = ax 2 + bx + c We could try to factorise or use other methods, but it is better to avoid these techniques during exams. Here, I will explain the solution to this quadratic inequality in a few logical steps.ġ) Firstly, we need to solve the quadratic equation by using the quadratic formula. It requires an understanding of the quadratic formula, as well as an understanding of substitution and the ability to sketch graphs. Unfortunately, there are no two ways about it: pupils dislike sketching graphs. In this article I am solving question nineteen of the June 2017 paper 3 (higher tier). Solving a GCSE Maths quadratic inequality question Parabola often feature in real world problems in economics, physics and engineering.Ī quadratic inequality is a second-degree equation that uses an inequality sign instead of an equal sign.

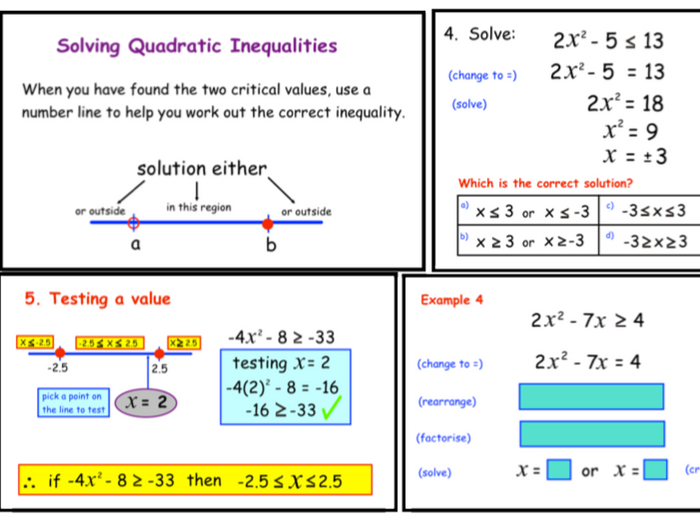
Quadratic equations describe parabolic motion: a symmetrical plane curve that can be drawn in the shape of a U. Let’s take a look at the expectations of the new GCSE maths curriculum by exploring a recently-introduced topic that pupils often struggle with: quadratic inequalities. This motivated them to introduce new concepts and focus more on developing reasoning skills rather than just calculation The British government wanted to bring the UK Maths GCSE in line with international standards and the demands of a changing job market. Look at the following examples, where we apply this process to solve quadratic inequalities.In September 2015, the GCSE Maths curriculum was updated to include new topics, including vectors, iterative methods and how to solve quadratic inequalities. We can accomplish this by using the graph or the statements from step 3. Step 4: Determine the inequality symbols that will make the solutions found in step 2 satisfy the inequality. Values above the x-axis are greater than 0, and values below the x-axis are less than 0.When the quadratic term is negative, the parabola opens down.When the quadratic term is positive, the parabola opens up and is U-shaped.Step 3: Obtain a simple graph of the function $latex y=ax^2+bx+c$, or alternatively, consider the following: We can solve this by factoring the quadratic expression. These are the points where the function $latex y=ax^2+bx+c$ intersects the x-axis. Step 2: Find the values of x such that $latex ax^2+bx+c=0$. Note: The “>” sign will depend on the problem. Step 1: If it isn’t, write the inequality in the form $latex ax^2+bx+c>0$. We can solve quadratic inequalities by following these steps:

The following are examples of quadratic inequalities: Quadratic inequalities are quadratic expressions that use inequality signs to compare two quantities.


 0 kommentar(er)
0 kommentar(er)
